34.5 Example: Removal efficiency

In wastewater treatment facilities, air from biofiltration is passed through a membrane and dissolved in water, and is transformed into harmless byproducts. The removal efficiency \(y\) (in %) may depend on the inlet temperature (in \(^\circ\)C; \(x\)).
The RQ is
In treating biofiltation wastewater, is the removal efficiency associated with the inlet temperature?
The population parameter is \(\rho\), the correlation between the removal efficiency and inlet temperature.
A scatterplot of \(n=32\) samples (Fig. 34.9) suggests an approximately linear relationship (Chitwood and Devinny 2001; Devore and Berk 2007). The output (jamovi: Fig. 34.10; SPSS: Fig. 34.11) shows that the sample correlation coefficient is \(r=0.891\), and so \(R^2 = (0.891)^2 = 79.4\)%. This means that about 79.4% of the variation in removal efficiency can be explained by knowing the inlet temperature.
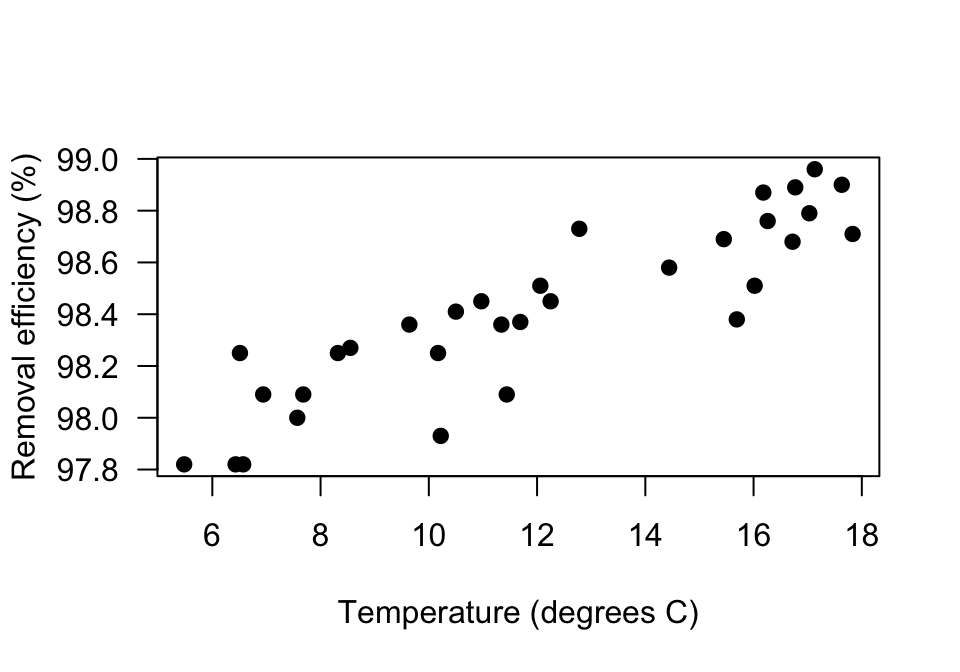
FIGURE 34.9: The relationship between removal efficiency and inlet temperature
To test if a relationship exists in the population, write:
- \(H_0\): \(\rho = 0\);
- \(H_1\): \(\rho \ne 0\): Two-tailed (as implied by the RQ).
The software output (jamovi: Fig. 34.10; SPSS: Fig. 34.11) shows that \(P < 0.001\) (which is what \(P = 0.000\) in SPSS means). We conclude:
The sample presents very strong evidence (two-tailed \(P < 0.001\)) that removal efficiency depends on the inlet temperature (\(r = 0.891\); \(n = 32\)) in the population.
The relationship is approximately linear and there is no obvious non-constant variance, and the sample size is larger than 25, so the hypothesis test results will be statistically valid.
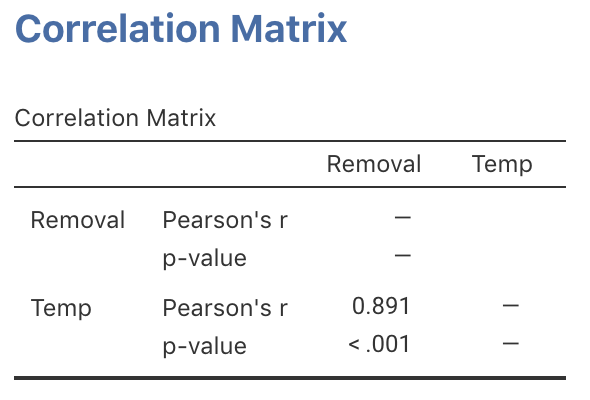
FIGURE 34.10: jamovi output for the removal-efficiency data
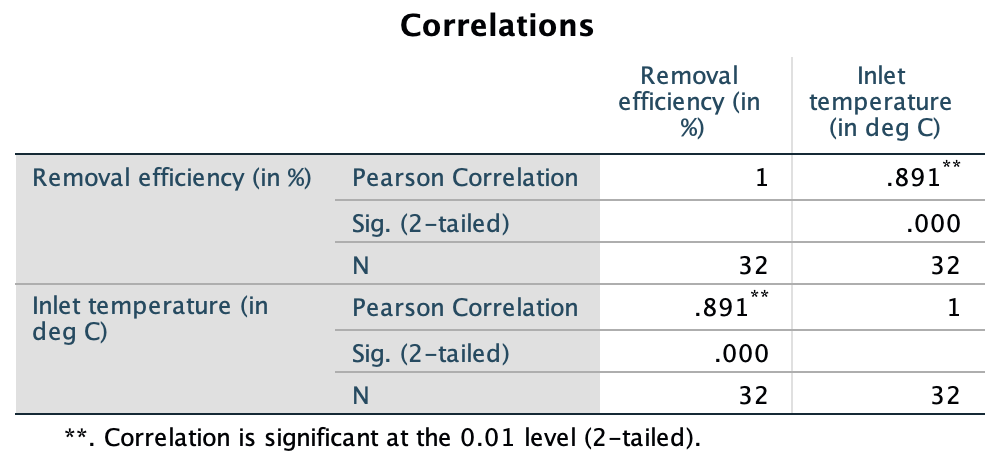
FIGURE 34.11: SPSS output for the removal-efficiency data