3.2 CDM Notations

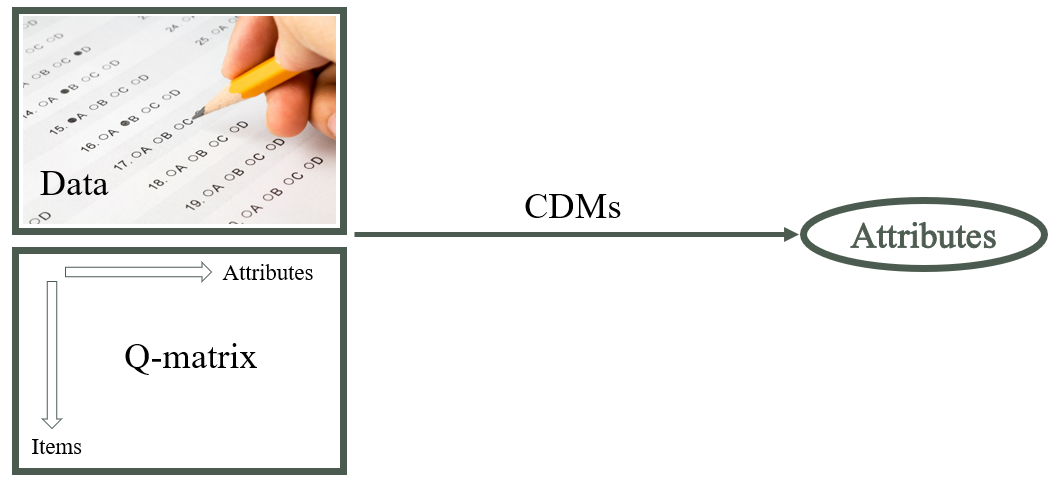
The response vector of examinee $i$ will be denoted by $Y_i, i =1, 2, ..., N$

The response vector contains $J$ items, as in, $\mathbf{Y}_i=(Y_{i1},\ldots,Y_{ij},\ldots,Y_{iJ})$

The attribute profile of examinee $i$ will be denoted by $\alpha_i=(\alpha_{i1},\ldots,\alpha_{iK})$

Each attribute vector or profile defines a unique latent class. For latent class $c$, we have

$$\alpha_c=(\alpha_{c1},\ldots,\alpha_{cK})$$

Thus, $K$ attributes define $2^K$ latent classes