2.11 Variation of flow through the tidal cycle

Pilots will often only give a flow speed at springs, leaving us with the factors method as the only option for estimating flow speed at other tidal ranges. When spring and neap rates are quoted, interpolation can also be used.

Another problem with pilots is that they typically only provide data on when the direction of flow changes (slack water) and what the maximum flow is. We may wish to estimate flow at other times. Two rules of thumb exist to do this – the 50/90 rule and the rule of thirds.

2.11.1 50/90 rule

The 50/90 rule states that:

We expect zero flow speed as the tidal stream changes direction (slack water)
One hour later, the flow attains 50% of maximum speed
Two hours after slack water, the flow attains 90% maximum speed
Three hours after slack water, the flow speed is a maximum (100%)
90% at 4 hours
50% at 5 hours
Slack water occurs again after 6 hours

It’s fairly obvious that the flow is zero at slack water and maximal 3 hours later. And it’s intuitive that the flow speed decreases in hour 4 and 5 the same way it increased in hours 1 and 2, so the main point to remember is:

50/90 rule: flow attains 50% of max speed after 1 hour, 90% after 2 hours

2.11.2 Rule of thirds

The rule of thirds is used to estimate average tidal flow speed during each hour. This makes it less useful in estimating flow at a given time, but more helpful if we are trying to estimate average speeds of paddling with tidal assistance (or hindrance). It states that:

During the first hour after slack water, average flow is 1/3 of maximum
During the second hour, average flow is 2/3 maximum
During the third and fourth hours, average flow is equal to the maximum
During the fifth hour, 2/3 maximum
During the sixth hour, 1/3 maximum

Again, it’s fairly easy to remember that flow is at a maximum during the third and fourth hours and that the flow speed decreases in hour 5 and 6 the same way it increased in hours 1 and 2, so the main point to remember is:

Rule of thirds: the average flow is 1/3 of max speed in the first hour of the tide, 2/3 in the second hour

Let’s look at an example:

Estimate how the tide will vary in Saint Mary’s Sound on 1 June 2020

The pilot tells us:

St Mary’s Sound: The east going stream begins at 5 hours before high water at Plymouth (Devonport). The west going stream begins at 2 hours after high water at Plymouth (Devonport). The flow reaches a speed of 1.7 knots at springs.

Consulting the Plymouth tide table….

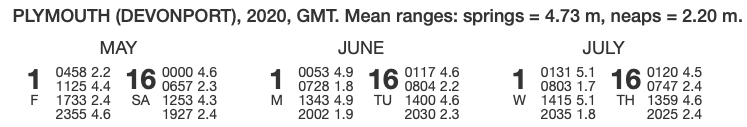
First row of tide table for Plymouth

The tide times at Plymouth on June 1st are:

01:53 BST 4.9 m  HW
08:28 BST 1.8 m  LW
14:43 BST 4.9 m  HW
21:02 BST 1.9 m  LW

As we’ve previously calculated: the tidal range is 4.9 m - 1.8 m= 3.1 m, giving a factor of 3.1 m / 4.73 m = 0.65, so maximum flow on June 1st = 0.65 X 1.7 knots = 1.1 knots.

Looking now at the afternoon high water, and recalling the pilot tells us that “The east going stream begins at 5 hours before high water at Plymouth (Devonport). The west going stream begins at 2 hours after high water at Plymouth (Devonport)”:

14:43 BST 4.9 m  HW - 5 hrs = 09:43 : E going stream starts
14:43 BST 4.9 m  HW + 2 hrs = 16:43 : W going stream starts

So, the east going stream will begin at 14:43 -5:00 = 09:43 and run until the west-going stream starts at 14:43 +2:00 = 16:43. We use the 50/90 rule to estimate the flow speeds. Unfortunately, the flow here runs for 7 hours, so we modify the rule slightly with 2 hours of 100% flow rather than one:

Time	Compared to slack water	50/90 rule	Flow speed	Direction
09:43	0	0%	0	-
10:43	+1	50%	0.6 kt	E
11:43	+2	90%	1.0 kt	E
12:43	+3	100%	1.1 kt	E
13:43	+4	100%	1.1 kt	E
14:43	+5	90%	1.0 kt	E
15:43	+6	50%	0.6 kt	E
16:43	0	0%	0	-