Epilogue
“Always be a little improbable” -Oscar Wilde
Academic Resources
Blitzstein and Hwang (2014): a masterful Probability textbook; the model for this book, which we have cited many times.
This channel provides Professor Joe Blitzstein’s Stat 110 lectures (again, Stat 110 was the fodder for this book).
Probability Cheatsheet: written by William Chen and Joe Blitzstein, a compact guide to probability theory.
Blyth (2013): an elegant, modern textbook on Statistics in Quantitative Finance.
Taylor and Karlin (1998): compact, yet thorough review of Probability and Stochastic Processes.
DeGroot and Schervish (2012): widely used textbook covering Probability and Inferential Statistics.
Dobrow (2016): a thorough introduction to Stochastic Processes, including practical simulations in R.
Rosenthal (2008): an intuitive, streamlined exploration of the Monty Hall problem
Teetor (2011): an extensive, well-organized and easy to read manual on using R.
Statistics in the World
538: the gold standard for Statistics in the media. Insightful data analysis on Sports, Politics, Economics, etc.
Lewis (2004): the national sensation (Moneyball) that discusses the rise of analytics in sports; also a major motion picture (2011)
Anderson and Sally (2013): soccer’s version of ‘Moneyball.’
Tango, Lichtman, and Dolphin (2007): intuitive discussions on using basic statistical analysis in baseball.
Lowenstein and (Firm) (2000): account of Long Term Capital Management, a hedge fund that enjoyed unprecedented success until it collapsed and nearly took down Wall Street’s biggest banks with it.
Lewis (2011): also a critically acclaimed movie (2015), chronicles the story of traders that anticipated the financial crisis of 2007-2008.
Sloan Conference: An annual, high-profile Sports Analytics conference held at MIT.
Taleb (2009): entertaining and holistic approach to the use (and misuse) of Probability in the world.
Vigen (2015): a lighthearted reminder of the dangers of confusing correlation and causation.
The movie “21” (2008) covers the rise and fall of the MIT Blackjack Team and even dramatizes a discussion of the Monty Hall problem.
Adams (2010): A humorous discussion of probability, improbability and, of course, the guide to the Universe!
Probability Playlist
If you’re going to learn probability, you have to do it with the right soundtrack.
Lotus (Cage the Elephant). A song that is either about a flower or an easily generalizable, multi-dimensional approach to finding expectations.
Changes (David Bowie). A musical adaptation of the transformation theorem for random variables.
House of the Rising Sun (The Animals). The singer discusses how his father was a “Gambling man,” and even hints at the old man having a ‘ruin.’
A Moment Like This (Kelly Clarkson). The first winner of American Idol probably wrote this song after learning about MGFs.
Across the Universe (Originally by The Beatles). As this song takes the listener across the universe, so does the Uniform distribution span the world of random variables via Universality.
Birthday (Katy Perry). If there are 23 people in a room, there’s a good chance that more than one of them knows this song!
Tomorrow (Annie). Weather is a often used as an example of a Markov process, and in this song Annie confidently gives her prediction for the state of the chain in the next round (tomorrow).
Final Words
In the academic world (and, in many senses, the modern world in general) we place a high premium on generating new ideas. Individuals are often measured by their novel contributions to a field. Some conduct research that unearths a new understanding about a topic; others complete an exceptionally difficult proof. Some reach these achievements through years of hard work and dedication; others from a singular moment of genius.
Consider how this ‘idea generation’ takes place. Most would likely argue for this type of model:
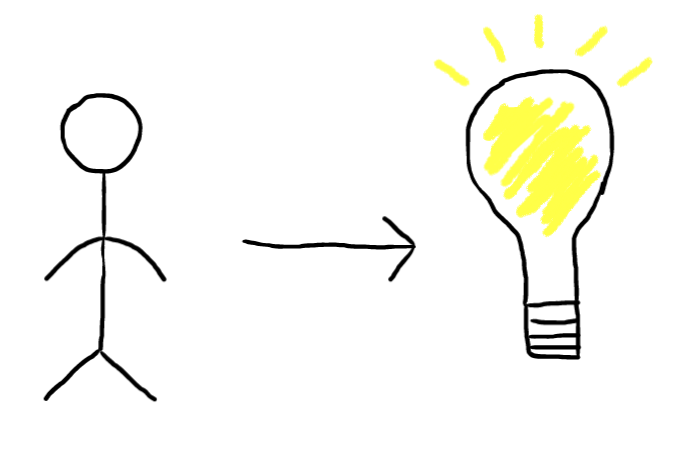
Here, we have an individual creating their dazzling contribution (the light-bulb). In fact, this is likely how many of us would capture the ‘generating’ process: a single brilliant person who works to create something special. However, in reality, this is a superficial model that only functions in a vacuum. We can zoom out for a more complete picture:
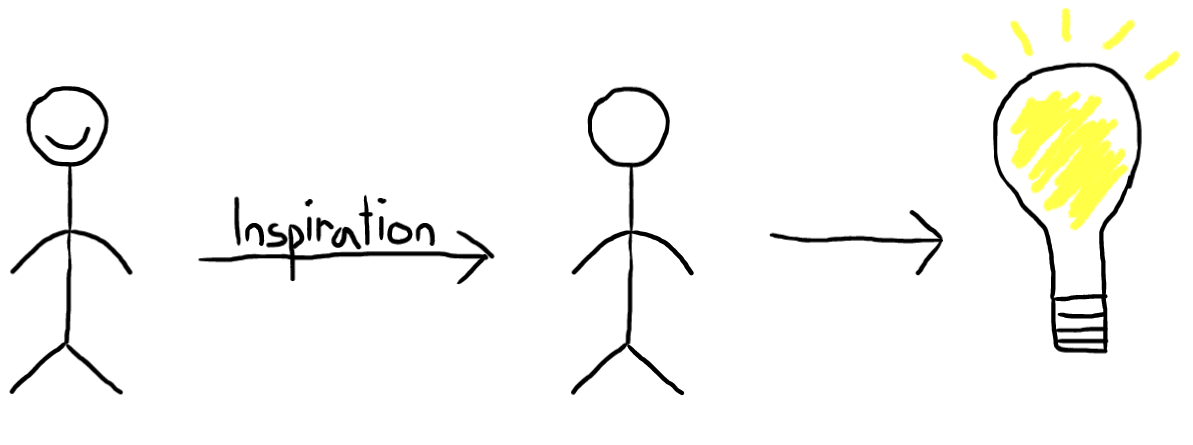
The point of this diagram is that, no matter how intellectual, hard-working, or creative an individual is, and no matter how much potential they have within a certain field, they have to be inspired first. Albert Einstein would have never become a worldwide phenomenon if he didn’t learn to love physics; Michael Jordan might be selling insurance if he didn’t find passion in the game of basketball.
This inspiration can come from anywhere, and it usually comes from someone else. If you could ask Einstein or MJ, they would probably be able to point a specific person that gave them an inspiration to engage in their field. Undergraduate institutions across the country constantly mix up these diagrams. Professors, TAs, etc. are too often focused on their own idea generation (the first diagram) that they forget to inspire the students around them; students that then, in turn, could go on to enhance the field!
The point here is that, no matter what you are passionate about (and, hopefully, that now includes Statistics), don’t forget to inspire others. Everyone has a role in their own field, and while it’s incredibly important to provide your own contributions, it’s just as valuable to inspire others to achieve themselves. Propagating passion, in any field, is always the first step.
After all, conditioning may be the soul of Statistics, but you are its worldly vessel!