1.12 Solution to the Additional Problems

1. First partial derivatives: $$\begin{align*} \frac{\partial E^2}{\partial A} &= \int^{30}_3\frac{\partial}{\partial A}(6.1(x+5.0)e^{-0.045x}-Ax-B)^2dx \\ &= 2 \int^{30}_3(6.1(x+5.0)e^{-0.045x}-Ax-B)(-x)dx \end{align*}$$ Equating to zero and dividing by 2 gives $$0 = -\int^{30}_36.1(x-2+5x)e^{-0.045x}dx +\int^{30}_3Ax^2+Bxdx$$ Successive integration by parts (for the first integral) and direct integration (for the second integral) yield the first equation: $$0 = 8991.0A + 445.5B - 25984.9$$ $$\begin{align*} \frac{\partial E^2}{\partial B} &= \int^{30}_3\frac{\partial}{\partial B}(6.1(x+5.0)e^{-0.045x}-Ax-B)^2dx \\ &= -2 \int^{30}_3(6.1(x+5.0)e^{-0.045x}-Ax-B)dx \end{align*}$$

Equating to zero, dividing by 2 and solving yields the second equation: $$0 = 445.5A + 27.0B - 1568.8$$

The solutions are $A= 0.061$, $B = 57.1$. The least squares integral using the approximation $Y = 0.061x + 57.1$ is 546.452.

1. Let $x=a\sin\theta$. The limits of integration are then changed as follows $$-a \le x \le a$$ $$-a \le a\sin\theta \le a$$ $$-1 \le \sin\theta \le 1$$

There are many options for the range of $\theta$. We first change the integrand to see which range is suitable. We know the integrand is non-negative since $x^2 \le a^2$. Substitution gives $$x = a\sin\theta$$ $$dx = a\cos\theta\:d\theta$$ $$\sqrt{a^2-x^2}dx = \sqrt{a^2 - a^2\sin^2\theta}\cdot a\cos\theta \:d\theta$$ The integrand is then non-negative when the positive square root is used and when $\cos \theta$ is non-negative. Thus, we choose the limits of integration to be $-\frac{\pi}{2}$, $\frac{\pi}{2}$ since $\sin(-\frac{\pi}{2}) = 1, \sin(\frac{\pi}{2}=1)$ and $\cos\theta \le0$ if $-\frac{\pi}{2} \le \theta \le \frac{\pi}{2}$.
The integral is then $$\int^{\pi/2}_{-\pi/2}\sqrt{a^2-a^2\sin^2\theta}a\cos\theta \:d\theta = a^2\int^{\pi/2}_{-\pi/2}\sqrt{1-\sin^2\theta}\cos\theta\:d\theta$$ $$\begin{align*} &= a^2\int^{\pi/2}_{-\pi/2}\cos^2\theta\:d\theta=a^2[\frac{\theta}{2}+\frac{\sin2\theta}{4}]^{\pi/2}_{-\pi/2} \\ &= a^2[\pi/4+(1/4)(0)-(-\pi/4)-(1/4)(0)] =\pi a^2/2 \end{align*}$$ Thus $$A=\frac{2b}{a}\int^a_{-a}\sqrt{a^2-x^2}dx = \pi ab$$ When $a = b = 5.57$, $A = 97.47$.
When $a = 5$, $b = 6.09$, $A = 95.66$.

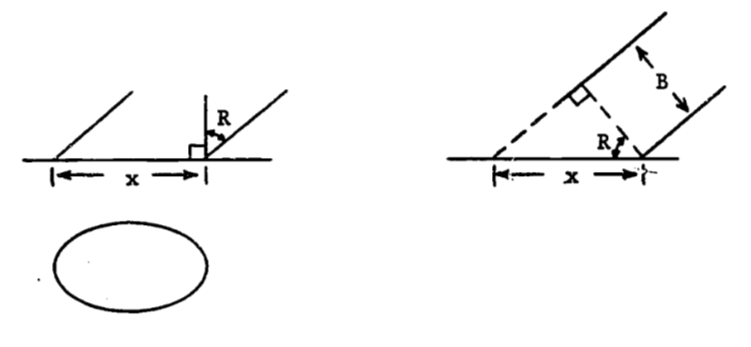
From the right-hand diagram, we have $$\cos R = B/x$$ and thus $$x=B/\cos R$$ The minor axis is then the circle’s diameter, $B$. In the answer to part “a”, we replace $2a$ by $B/\cos R$ and $2b$ by $B$.

$$\begin{align*} A &= \pi ab = \pi(B/2\cos R)(B/2) \\ &= \pi B^2 / (4 \cos R) \end{align*}$$

Since the area of the circle is $\pi B^2/4$,the dilution coefficient is $$D = \frac{\pi B^2/4}{\pi B^2/4\cos R} = \cos R$$ Thus $D=0.6$ means less dilution than $D=0.3$, and $D=1.0$ means no dilution at all. For Minnesota, the coefficients are
$D=0.94$ for $R=20°$
$D=0.42$ for $R=65°$