1.10 Reversing the Condition
Medical tests used to diagnose a condition in a patient are rarely, if ever, 100% reliable. For a patient who either has or does not have the condition, the test will come back with the result either report positive or negative.
For any positive result, the patient may either really have the condition (a true positive) or not actually have the condition (a false positive). For any negative result they may either really not have the condition (a true negative) or actually have the condition (a false negative). This can be summed up as:
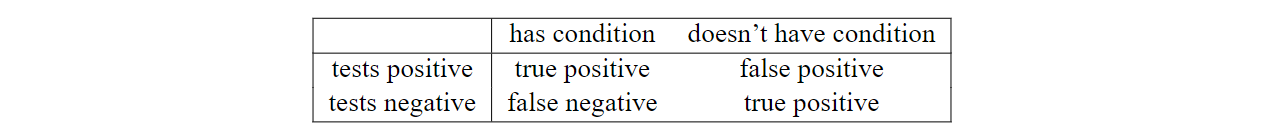
It is important for health professionals to understanding how to interpret tests results appropriately, with three key variables needed to do so correctly:
Sensitivity - the of patients with the condition correctly given a +ve result, or \(P(\text{positive | condition})\).
Specificity - the of patients without the condition correctly given a -ve result, or \(P(\text{negative | }\overline{\text{condition}})\).
Prevalance - the proportion of individuals in the population that actually have the condition, or \(P(\text{condition})\).
If a patient tests positive for a condition the question will be relevant to them is: “Given that I tests positive, what is the probability that I actually do have the condition? Here, \(P(\text{positive | condition})\) is known and the patient wishes to know \(P(\text{condition | positive})\). This can be seen as a case of the more general problem of reversing the condition. In other words, if \(P(A|B)\) is known, how can \(P(B|A)\) be calculated?
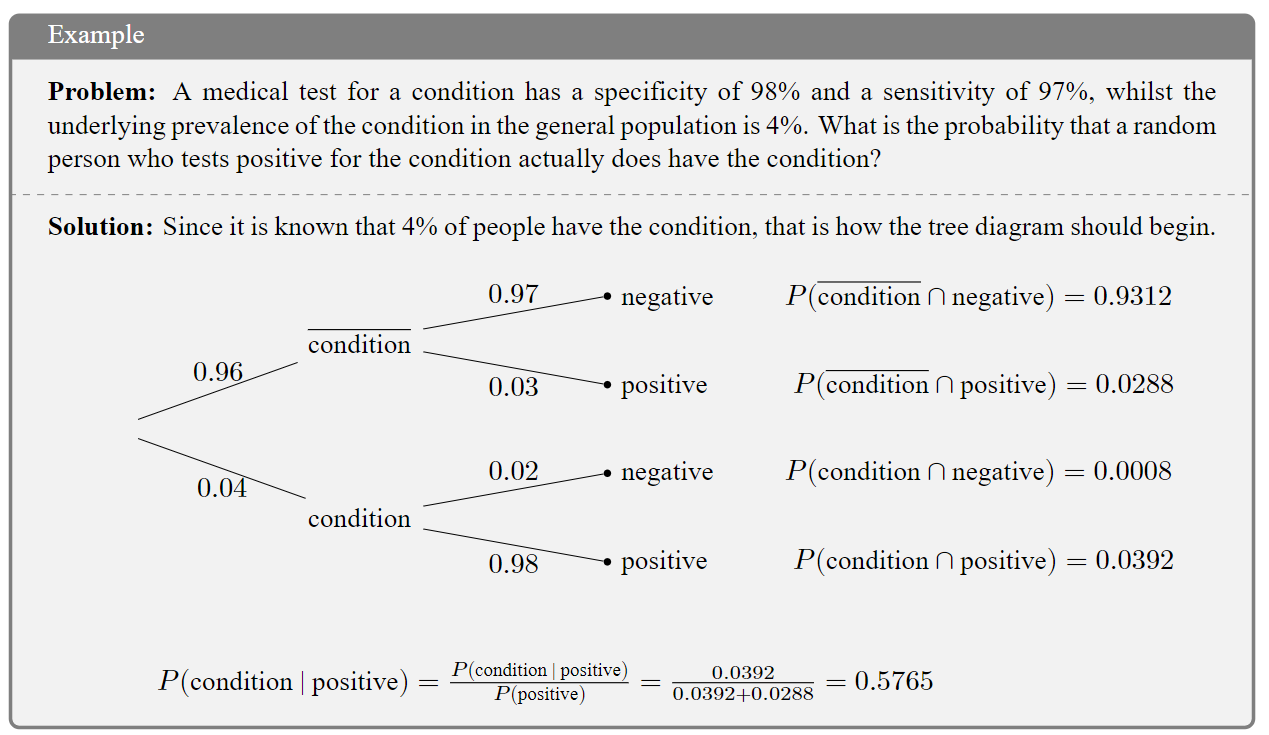
It may be surprising that a test which appears so effective can lead to such a high proportion of false positives, and calculations such as this should be considered when healthcare professionals establish testing procedures. In practice, the false positive rate can be drastically reduced by deciding when a test may be appropriate. GPs for example will ask questions about a patient’s symptoms and medical history to determine whether they have reasons to suspect that the chance that they have the condition is significantly greater than a random member of the population.