1.11 Bayes Theorem
In 1763 the Reverend Thomas Bayes, also a statistician, published “An Essay towards solving a Problem in the Doctrine of Chances”. In it, he set out both a formula that has come to be known as Bayes’ Theorem as well as an approach to thinking about combining existing knowledge with new information that can be referred to as Bayesian thinking. In turn, this thinking lead to an approach towards statistcal inference that has been growing since the mid-20th century called Bayesian statistics, substantially made more feasable bu modern computing power.
Note: Whilst knowledge of Bayes’ Theorem is indicated as part of the Advanced Higher Statistics course specification, Bayesian statistics or even Bayesian thinking are not part of this course, and so will not be covered here. If you would like to find out what these are and whether they may be relevant to you in the future, it is recommended that you discuss this with your teacher at a later point in the course, after gaining a thorough understanding of hypothesis testing. Modules introducing these ideas are typically available in the second or third years an undergraduate degree in Mathematics or Statistics.
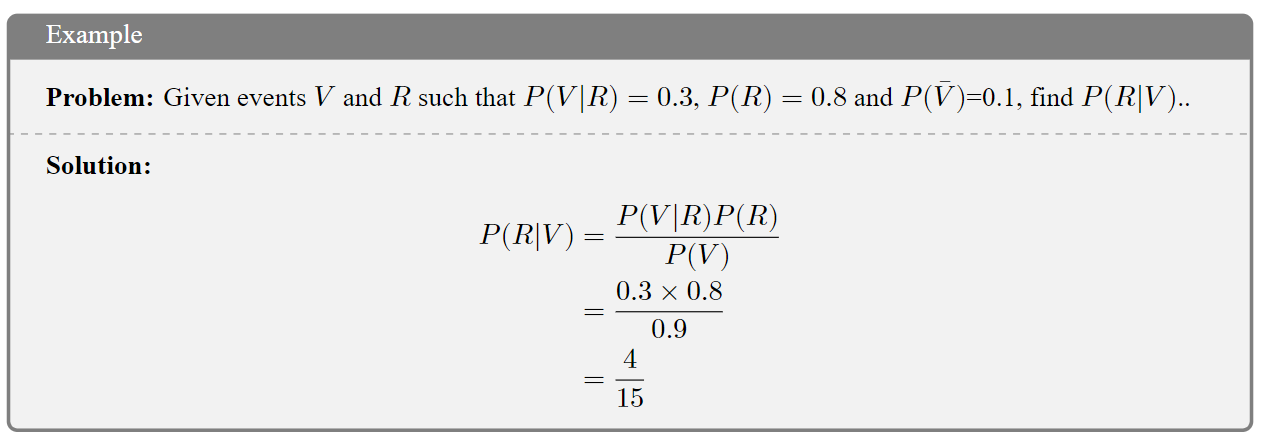
Bayes’ Theorem itself is easily derived by remembering that \(P(A\cap B)=P(B\cap A)\) and equating the multiplication rules for each, then rearranging to give:
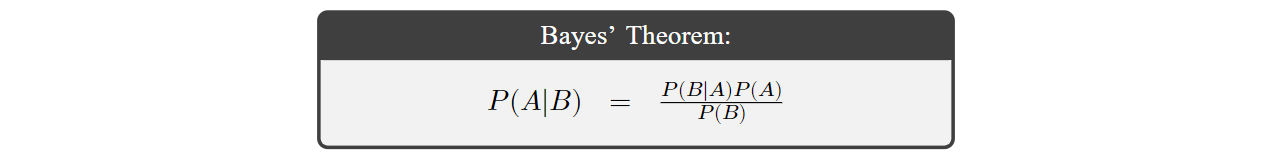
The reason for the shortness of this section is that any probability problem for which Bayes’ Theorem may be used can also be solved often more simply using a tree diagram and the rule for conditional probability. Nevertheless, you should be aware of the formula and it may be of use.