1.8 Independent Events
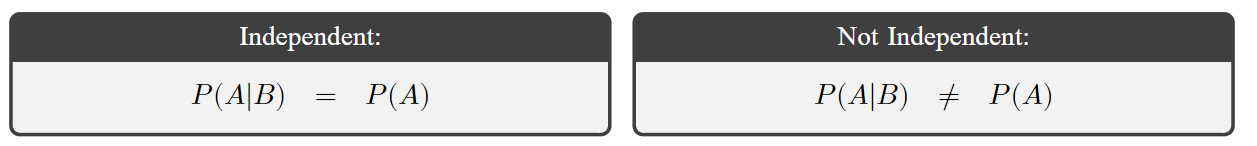
The probability of tossing a coin and it landing tails up is \(\frac{1}{2}\). The probability of it landing tails up given that it is a weekday, notated \(P(\text{tails }|\text{ weekday})\) is still \(\frac{1}{2}\). Since knowing that it is a weekday has no effect on the coin landing tails up, the two events are said to be independent of each other.
In comparison, the probability that a randomly selected person has an umbrella with them is likely to be different than the probability they have an umbrella given that it is a rainy day. Since knowing whether it is rainy changes the probability of them having an umbrella it is said that the two events are not independent of each other.
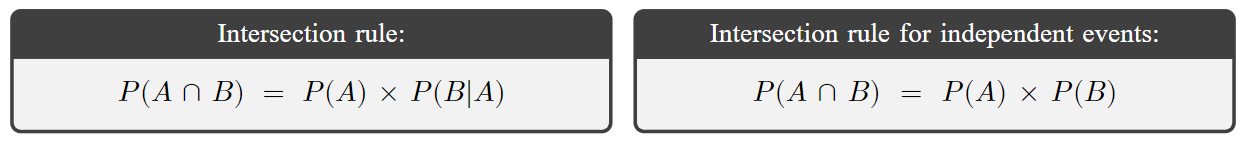
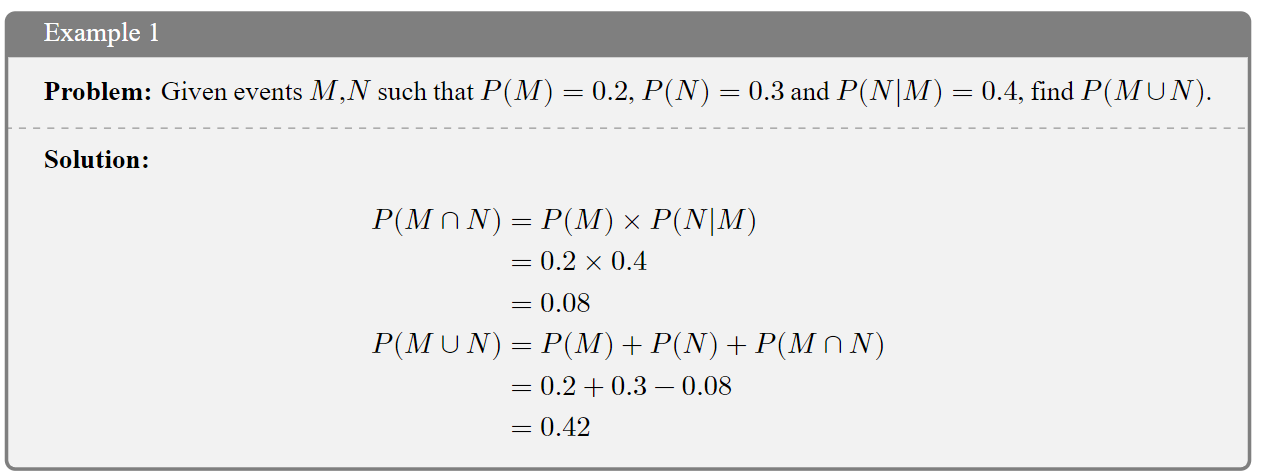
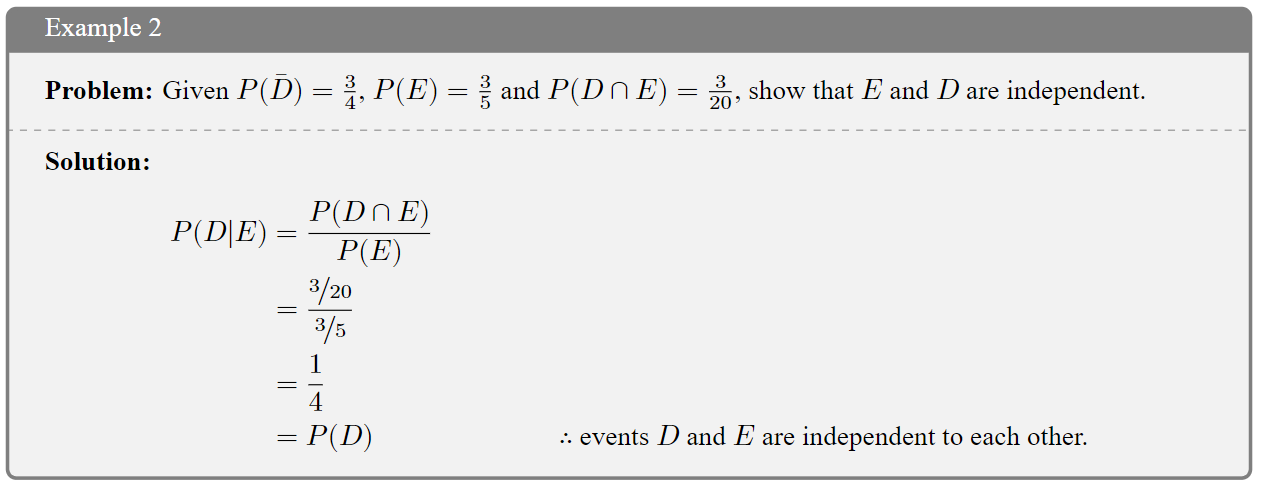
The conditional probability rule can be rearranged to allow the intersection of two events to be calculated using a conditional probability, and for independent events, since \(P(B|A)=P(B)\), this simplifies further.